Web Mercator Projection – One of Cylindrical Projections of an Ellipsoid to a Plane https://doi.org/10.32909/kg.20.35.2

Abstract

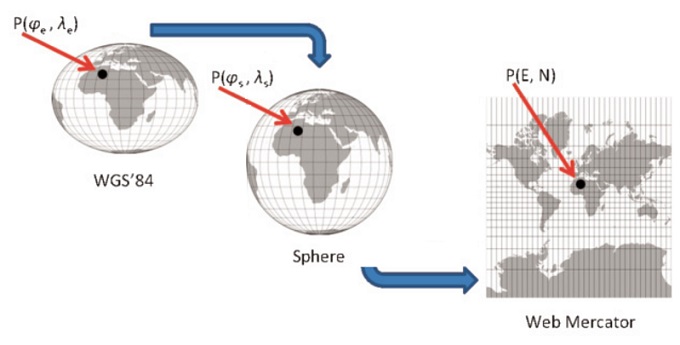
The Web Mercator projection is a projection of a relatively recent date. There has been a lot of controversy about its application. Some believe that this projection is not a projection of either the sphere or the surface of the ellipsoid. Therefore, in this paper, several projections of the surface of a rotational ellipsoid into a plane are investigated and it is shown that the Web Mercator projection is one of such projections. Namely, although the equations of this projection are identical to the equations for the projection of the sphere, the basic difference is in the choice of the area of definition, i.e., the domain of the projection. Furthermore, we have shown that the Web Mercator projection can also be interpreted as double mapping: mapping an ellipsoid to a sphere according to the normals and then mapping the sphere to the plane according to the formulas of the Mercator projection for the sphere. The Web Mercator projection is not a conformal projection, but it is close in properties to the Mercator projection.